Manfred Boergens - Mathematics on stamps - How to find South

Manfred Börgens
Mathematics on stamps

Gabon 1967 Michel 283 Scott C56

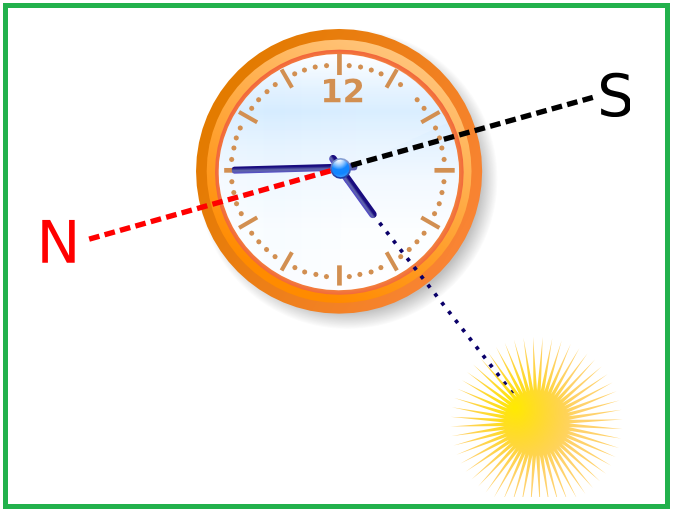
How to find South - Scouts' method

This stamp was issued to honour the 12th World Scout Jamboree at Farragut State Park in the Rocky Mountains, Idaho, USA. The Jamboree took place from July 31 to August 9, 1967 and welcomed 12000 scouts from 105 nations.

Scouts learn to cope with natural conditions in the open landscape. This includes knowledge of the motion of the sun, the moon and the stars. The stamp shows a method to find the geographic directions without a compass. The method employs a traditional watch with hands.

One could object that nowadays the orientation in the landscape is much facilitated by GPS devices. But being familiar with traditional methods has its merits because they provide understanding and curiosity about natural phenomena on earth and sky to young people. The question how observations made in our environment are connected and can be explained leads to acquiring basic scientific knowledge. This may be a good way to learn that mathematics, physics and astronomy can give pleasure (instead of ordeal). – It should be mentioned that many Waldorf schools' curricula include a hands-on training in surveying. This excursion into the countryside takes about one week and makes the students familiar with the basics and principles of geodesy and cartography. This is achieved in a markedly application-oriented manner and seems to me to be a commendable didactical way to teach scientific competences.

The scouts' method to find South

Back to the stamp shown above: The upper (white) part shows the orientation in daylight, the lower (blue) part shows the orientation at night, both for the northern hemisphere. Below, we will discuss in detail how to find South by using a watch and the position of the sun. But first to the blue part of the stamp: At night and when the sky is clear, one can easily find North by looking for the Polar Star. On the stamp, it is the leftmost star of the Little Bear (Ursa Minor, Little Dipper). In most parts of middle Europe, this constellation is nearly invisible, because of air and light pollution – with the exception of the bright Polar Star. In 1967, the scouts who had gathered in the Rocky Mountains could have seen the Little Bear.

The method shown on the stamp is also displayed in figure 1. How is this method applied? The stamp shows the time 14:17 , figure 1 shows 16:45 . In both cases the hour hand is turned to the direction of the sun - strictly speaking, to the point on the horizon perpendicular to the sun. South is the direction which bisects the angle between the hour hand and the 12 on the watch.

South
Figure 1 Finding South with a watch and the position of the sun Copyright

Now we know the scouts' method to find the geographic directions. But how exactly does it work? And is it a correct method?

First, we want to explain the simple and plausible idea behind the scouts' method. With the watch laid flat, the hour hand and its projection onto the horizon rotates evenly twice a day. The sun moves approximately along a circle, too – not along the horizon but above the horizon at day and below at night (this deviation will be ignored here and be discussed later). The sun moves along this circle once a day, his revolution is therefore only half as fast as the revolution of the hour hand. And that's all. That's the idea behind the scouts' method. At 12:00 , the sun stands in the South and the angle between sun and hour hand is 0° and needs not be bisected. In the afternoon the hour hand runs twice as fast as the sun, therefore the angle between the hour hand and the 12 on the watch must be halved to get the south direction. Before noon, this method works in analogous manner because the movement of the sun behaves symetrically to the noon (south) position. This can best be seen in figure 2 where the south direction is kept fixed.

Some readers may miss the remark that the revolution of the sun is only apparent to us and not happening in reality. The heliocentric system teaches that our perception of this revolution is caused by the rotation of the earth and not by the sun circling the earth once a day. But geometrically, both views – heliocentric and geocentric – are equivalent. In this article we consider only relative movements of earth and sun which do not allow for any discrimination in "true" or "false" between helio- and geocentric models. In many cases, the geocentric standpoint is much more advantageous for describing astronomical phenomena.

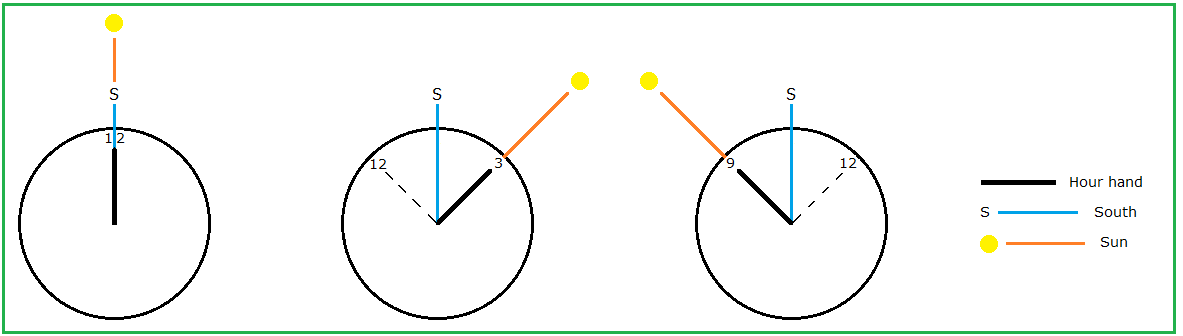
Sueden oben

Figure 2 Position of the sun and south direction at 12:00 , 15:00 and 09:00

Local time

We now know how to apply the scouts' method. But that's not the whole story. First of all, we should mention the necessary arrangements for the method to make sense.

Latitude The scouts' method relies on the sun standing in the south at noon. This is valid year-round only north of the Tropic of Cancer (23.4° North). For now, we will assume that the observer stays there. Other regions of the earth will be considered later.

Sun Time   The scouts' method is based on the position of the sun. Thus the watch must show True Sun Time. At 12:00 the sun should stand in south position (and at maximum height). The most important precondition is the knowledge of the observer's longitude and of the longitude for which the watch is normally set. The distance of neighbouring degrees of longitude corresponds to the time difference 4' (4 minutes).

Example: The scouts stay at their summer camp in the German Taunus mountains at 8° East. Their watches show the standard time UTC+2 (the legal German Summer Time including daylight saving time). This is the Mean Sun Time (that is the local Mean Time) for 30° East. Compared to the Mean Sun Time at 8° East we find a time difference of 88'. The scouts' watches are 1h 28' fast compared to their local Mean Sun Time. Thus the sun stands at 13:28 in the South (and not at 12:00 – this corresponds to the observation that old sundials near the scouts' camp are 1h 28' slow (on average) compared to the legal time because in former times sundials displayed local True Sun Time).

Why have we introduced the term Mean Sun Time in the last section – while the term we first used was True Sun Time ? The adjustment of the watch for local standard time and for longitude does not suffice for indicating the correct sun time - because the sun should stand in his south position at exactly 12:00 , but this only applies to the average over the year. The days are not of equal length but yearly fluctuate around 24 h - this is caused by the tilt of the earth axis and the elliptical shape of the earth orbit. The accumulation of these fluctuations leads to the Equation of Time. True Sun Time is the sum of Mean Sun Time and Equation of Time, it thus depends on the observer's position and the date. – The consequence for the scouts' method is that the watch must additionally be adjusted forth or back by some minutes, depending on the Equation of Time indicating positive or negative values (see chart).
During Easter and summer time, this adjustment can be dropped due to insignificance. But the adjustment is important from mid-January to mid-March and in October and November (see chart).

On the 1st of March, the Equation of Time shows -12', thus in our example above with 8° East the following calculation should be made:
     Mean Sun Time is slow by 7×4' = 28' compared to the watch. Keep in mind: On the 1st of March, daylight saving time is not yet effective; so the legal time zone is UTC+1.
     Due to the Equation of Time, True Sun Time is slow by 12' compared to Mean Sun Time.
     Hence the scouts have to adjust their watches by 40' (back) in order to obtain their local True Sun Time.

Symmetry a.m./p.m. The position of the sun shows a symmetry with respect to 12:00 noon . Before noon, the sun as pictured on the stamp would stand to the left of the South direction. Due to this symmetry, the scouts' method works before noon in an analogous manner as after noon (cf. right image in figure 2).

By studying figure 2 we can find yet another symmetry. Between 06:00 and 18:00 , we determine the South direction by bisecting the smaller angle between the hour hand and the 12 on the watch. If we would bisect the bigger angle we would get the North direction (cf. figure 1). The symmetry just mentioned becomes clear if it is earlier than 06:00 or later than 18:00 ; then South and North must be interchanged when using the scouts' method. Figure 1 shows this effect quite perfectly – you only have to imagine the time not being 16:45 (with the sun standing in a westerly direction) but 04:45 (with the sun standing in an easterly direction); then you will have to interchange South and North in the graphic.

Shortcoming of the scouts' method: Angle distortion

Up to this point, we only explained the necessary requirements for the application of the scouts' method. Do these provide sufficient certainty for determining the South direction with an acceptable precision? Unfortunately, this is not the case. Why can this method not work perfectly? Only if the sun moves along a circle roughly parallel to the horizon, this circle is roughly parallel to the face of the watch – in this case the idea described above concerning the double speed of the hour hand takes effect. But such a daily sun circle will only be witnessed in the polar region, so only there one can expect the scouts' method to work with an acceptable precision. In the mid-latitudes (e.g., in Europe and North America) one has to face major deviations.

Not only the latitude is crucial for the precision of the scouts' method but season also can lead to an error. The scouts' method implies that the sun reaches East at 06:00 and West at 18:00 . This is only true on spring and autumn equinox. It is easy to watch the substantial deviation from these directions during midsummer and midwinter.

We now will show the difference between true South and South as indicated by the scouts' method.

Computation of the angle in the scouts' method

τ   time measured in degrees: τ = 0° = 00:00 , τ = 90° = 06:00 etc. (True Sun Time)
      This is not the angle shown by the watch but half of it!

τ_o   time shown on the watch: τ_o = τ/15°

A   Azimuth (angle of the sun read along the horizon): A = 0° = North, A = 90° = East etc.

The middle and the right graphics in figure 2 show the increase of the angle between the North direction as assumed by the scout and the azimuth; it amounts to 15° per hour. (E.g., if in the right graphic in figure 2 we would take 10:00 instead of 09:00 the angle between the hour hand and the 12 on the watch would grow by 30° , thus the angle between the hour hand and the assumed South direction would grow by 15°.)
This yields a very simple formula for the scout when she or he assesses the direction of the sun:

(1)   A_Scout = τ_o·15° = τ

Computation of the angle for the true position of the sun

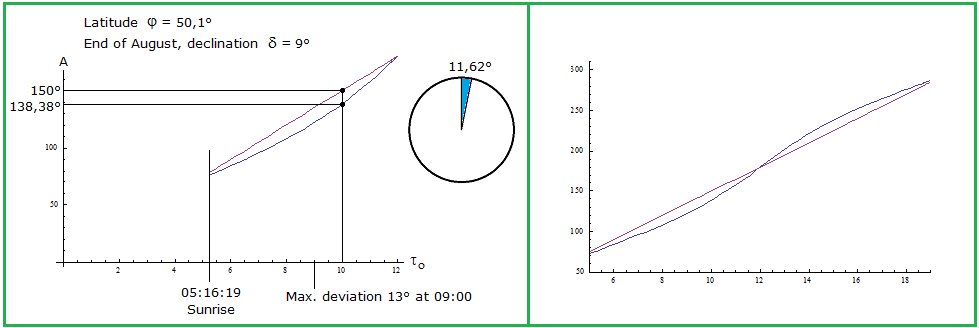
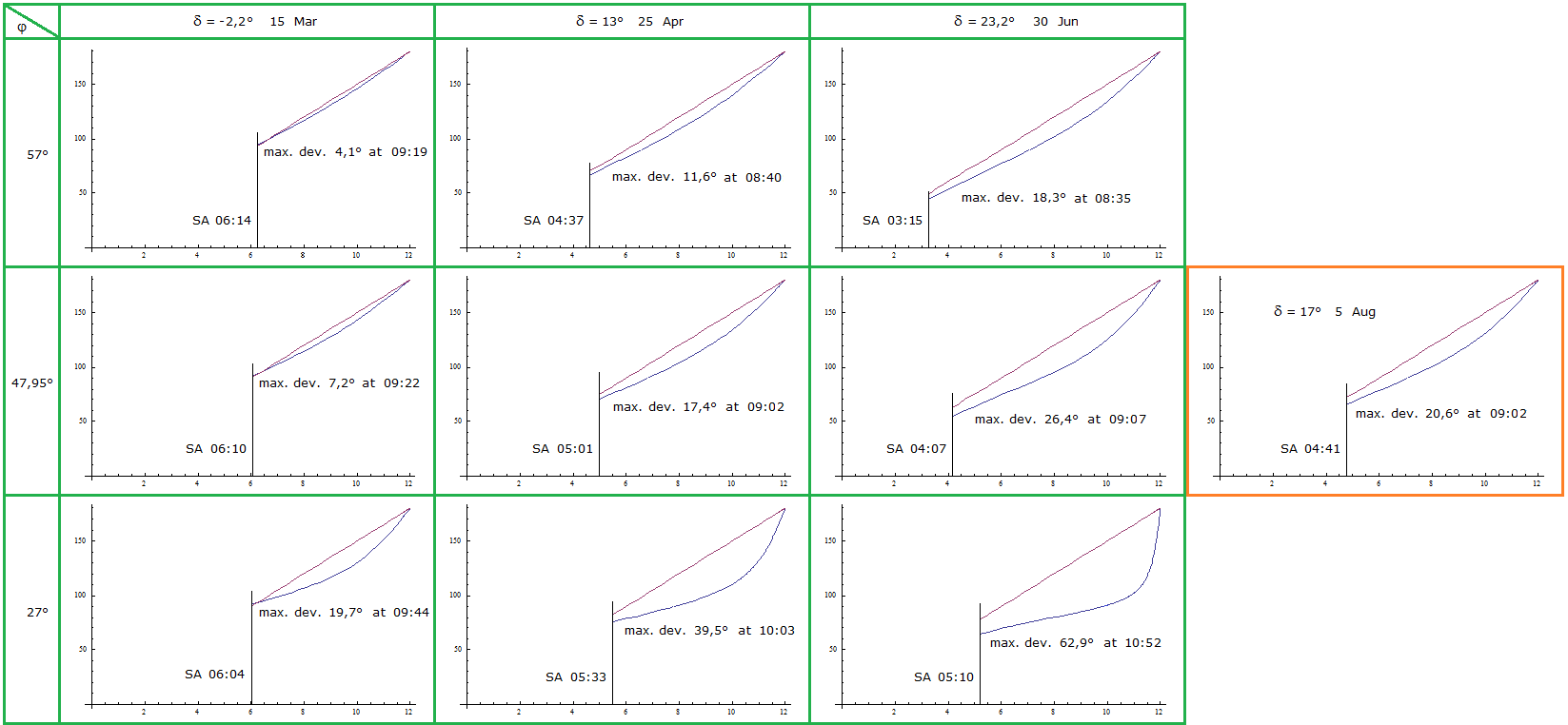
The true azimuth depends on three parameters: time of day, date and latitude. (The scouts' method only uses time.)

The date is usually replaced by the declination of the sun. This is the latitude where at noon the sun stands in the zenith. The translation from date to declination and back is made with a declination table.

τ   time measured in degrees: τ = 0° = 00:00 , τ = 90° = 06:00 etc. (True Sun Time)

δ   declination

φ   observer's latitude

Pertinent sources give different (but equivalent) formulae for the azimuth. These make simplifying assumptions (spherical shape of the earth, no atmospheric refraction) which in our context generate merely small and negligible deviations. We take the formulae deduced in Blog # 3, formula (14) on this website. Due to the symmetry a.m./p.m. explained above it is sufficient to compute A for the time before noon:

(2)   A_true = arccot(cot τ sin φ + csc τ tan δ cos φ)

Remark: The Mathematica definition of arccot is the inverse function of cot:[-π/2,π/2]\{0} → R , in contrast to the more familiar definition as the inverse function of cot:(0,π) → R , which we used in Blog # 3. If you want to compute A with Mathematica you should use the Mathematica function acot[x_]:= ArcCot[x] + If[x<0,π,0] instead of ArcCot[x] .

Furthermore, for A_true as well as for A_Scout we get: τ = 0° ⇔ A = 0° and τ = 180° ⇔ A = 180°.

At day time τ , we derive from (1) and (2) the difference between the South direction and the azimuth :
South_Scout = 180° - τ = 180° - A_Scout South_true = 180° - A_true

(3)   South_true - South_Scout = A_Scout - A_true

We shall see that this difference between true and assumed South direction can be considerable.

Sunrise

For the scouts' method being employed the sun must shine. The following graphics show the time between sunrise and noon on the horizontal axis. The time for sunrise was deduced in Blog # 3, formula (11). We display the formula as time of day (analogous to τ_o ) :

τ_rise = arccos(tan δ tan φ)/15°

A first instance of the imprecision of the scouts' method is shown in figure 3. We use again the example of the scouts' camp in the Taunus mountains at 8° East and (say) 50,1° North . The longitude is not relevant here because we use True Sun Time. The scouts meet during the summer holidays; we pick the declination δ = 9° corresponding to 31^st August (or 13^th April in the Easter holidays), cf. declination table .

In figure 3 the horizontal axis shows the time of day τ_o and the vertical axis the direction A . The red curve shows A_Scout and the blue curve shows A_true .

Taunus im August

Figure 3   In which direction A is the sun standing between sunrise and noon?
                Left: lower curve shows true direction A_true , upper curve shows direction according to scouts' method A_Scout
                Right: symmetry respective to 12:00

Figure 3 gives a first impression of the magnitude of the measurement error in mid-latitudes. At 10:00 (and also at 14:00), the scouts' method gives the South direction with an error of 11,62° (the circle on the right in the left graphic shows how big this angle is); at 09:00 (and at 15:00) the error would be 13°, this is the maximum error. (3) implies that before noon the true South lies more to the West and in the afternoon more to the East.

We shall see that in more southernly latitudes and in midsummer the measurement errors are even greater. But if you want to find a hidden treasure an error as big as in figure 3 is not negligible.

Figure 4 shows systematical effects of latitude and date upon the reliability of the scouts' method.

In the rows of figure 4 we show three different northern latitudes:

57° At this latitude, one will meet North American scouts rather rarely, with the exception of South Alaska. In Europe, this latitude goes through North Denmark and South Sweden, and in Scotland through Braemar and Mallaig.

47,95° This latitude refers to the stamp presented above because it is the latitude of Farragut State Park. In Europe, it goes through the centre of France and through South Germany.

27° This is a subtropical latitude going through Florida, Egypt and North India.

In the columns of figure 4, we show four different dates with the corresponding declinations:

15^th March This date stands as an example for the days around the equinoxes.

25^th April This could be a day in the Easter holidays.

30^th June This date stands as an example for the days around the summer solstice.

5^th August (for Farragut State Park only) On this day, the Jamboree mentioned on the stamp was in full swing.

Figure 4   In which direction stands the sun between sunrise and noon?
              Horizontal axis: Time of day  τ_o
              Vertical axis: Direction of the sun A (in degrees), measured from North in clockwise direction
              SA = Sunrise
              Lower curve: true direction. Upper curve: direction according to the scouts' method

Figure 4 displays the effects of latitude and declination quite well. With ascending latitude we see an improvement of the accuracy of the scouts' method. This was explained above: In high latitudes the sun takes a flatter orbit above the horizon, an orbit matching a watch held horizontally quite well. The accuracy also improves near the equinoxes when the declination of the sun ist small. The reason for this effect is that only at the equinoxes the sun runs along a perfect semi-circle between sunrise and sunset and only then stands in the East at 06:00 and in the West at 18:00.

In the subtropics the scouts' method will fail. Due to the date in midsummer this also applies to the Jamboree featuring on the stamp (orange box in figure 4). Regrettably the fine design of the stamp is therefore somewhat reduced in its didactical content.

In the North polar region, the scouts' method can be applied quite effectively, e.g. in Alaska and Scandinavia. Obviously, it does not work for δ ≤ φ - 90° (polar night). For δ > 90°- φ there is no sunrise because the sun shines all day; the scouts' method can be applied nonetheless.

The graphics in figure 4 also expose familiar rules for the sunrise times. At a certain place the rule holds: The greater the declination the earlier the sun rises. During wintertime (from the beginning of autumn until the beginning of spring, cf. left column in figure 4) the rule holds: The more northernly the observer is located the later the sun rises; it's the other way round in summertime.

Tropics und southern hemisphere

What has been said about the subtropics applies to an even bigger extent to the tropics: The scouts' method is unusable there. Due to the steep angle the path of the sun takes at sunrise and sunset the contortion of the orbit angles vs. the directions on the horizon is much too large. Even more important is the fact that in the tropic regions the sun at noon stands in the South at certain days and in the North at other days. This holds in particular for Gabon which is crossed by the equator. The stamp displayed above certainly has its merits for mathematical philately and for geomathematical didactics – but in the country which issued it the method shown on the stamp cannot be applied.

At last, to the region south of the Tropic of Capricorn ( φ < -23.4°) where by intuition one would apply all the rules we learned for the North "symmetrically". But this would only work with a watch whose hands move counterclockwise. For φ < δ the sun moves from East to North to West, thus from right to left. One can use a smart modification of the scouts' method published by the South African scouts: You have to turn the 12 on your watch to the direction of the sun and then to bisect the angle to the hour hand. One can memorize this symmetry in the scouts' method with respect to northern and southern latitudes in the following way: Swap North and South, swap hour hand and 12.

Published 2021-10-25 last update 2021-01-18

stamp list | previous stamp

Manfred Börgens | main page